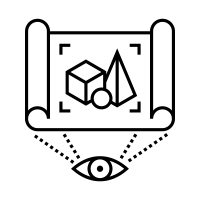
The tangent line on a point of the curve is the straight line (linear function) that runs through that point and has the same slope as the curve at this point. While the tangent line can be computed by calculating the slope on each point, this is “luckily” a direct product of our derivative f’(x) :
$$tangent_{line}(x) = f'(a) (x-a) + f(a)$$

The linear equation of the tangent line can be read at the following :
$$tangent_{line}(x) = ax + b$$ The constant a is f’(a): the slope of the curve at point A.
The constant b is f(a): the value of the curve at point A (its offset).
The variable x is (x - a): we just moved the abscissa origin of the tangent line to the point A (cf. translation).