Multiplying or dividing fractions is just as simple as a multiplication. To multiply fractions, we simply work straight across them : we multiply the numerators together and the denominators together. It is that simple !

Example

$$\frac{2}{5} × \frac{1}{3} = \frac{2*1}{5*3} = \frac{2}{15}$$

When we add or subtract fractions, fractions must have the same denominators; for multiplications and divisions, it doesn't matter.

Simplification

Remember to simplify the fractions first in order to make the problem easier to perform.

For instance: $$\require{cancel} \frac{3}{15} × \frac{2}{6} = \frac{\cancel{3} \cdot 1}{\cancel{3} \cdot 5} * \frac{\cancel{2} \cdot 1}{\cancel{2} \cdot 3} = \frac{1}{5} * \frac{1}{3}$$ It is easy calculate $$\frac{1}{5} * \frac{1}{3} = \frac{1 * 1}{5 * 3} = \frac{1}{15}$$ While it is much more difficult to perform $$\frac{3}{15} × \frac{2}{6} = \frac{3 * 2}{15 * 6} = \frac{6}{90} = \frac{\cancel{6} \cdot 1}{\cancel{6} \cdot 15} = \frac{1}{15}$$

Then, also remember to simplify the resulting fraction if it needs to be.

Watch the Negative Signs

Make sure you carry those negative signs through your calculations.

Remember that the minus sign can be moved all over a fraction: $$\frac{-a}{b}=-\frac{a}{b}=\frac{a}{-b}$$ $$\frac{-a}{-b}=\frac{a}{b}$$

Example

$$\frac{-2}{5} × \frac{1}{-3} = \frac{-2*1}{-5*3} = \frac{-2}{-15} = \frac{2}{15}$$

If we know how to multiply by fractions, we already know how to divide by fractions. Just flip the second fraction upside down and multiply instead dividing.

Dividing by 1/4.

Is the same as multiplying by 4/1.

So if we have: $$\frac{1}{2} / \frac{1}{4}$$ Which is the same as writing: $$\frac{1}{2} / \frac{1}{4} = \frac{\frac{1}{2}}{\frac{1}{4}}$$

We procceed the inversions: $$\frac{1}{2} / \frac{1}{4} = \frac{1}{2} * \frac{4}{1}$$ Then: $$\frac{1}{2} * \frac{4}{1} = \frac{1 * 4}{2 * 1} = \frac{4}{2} = 2$$

That's it. We now master how to multiply and divide all fractions!

Reminder

The number above, at the numerator, represents the number of shares we take.
The number below, at the denominator, represents the number of total shares.

We never divide by 0!

Multiplying

Why is the solution a smaller number than the fractions involved?

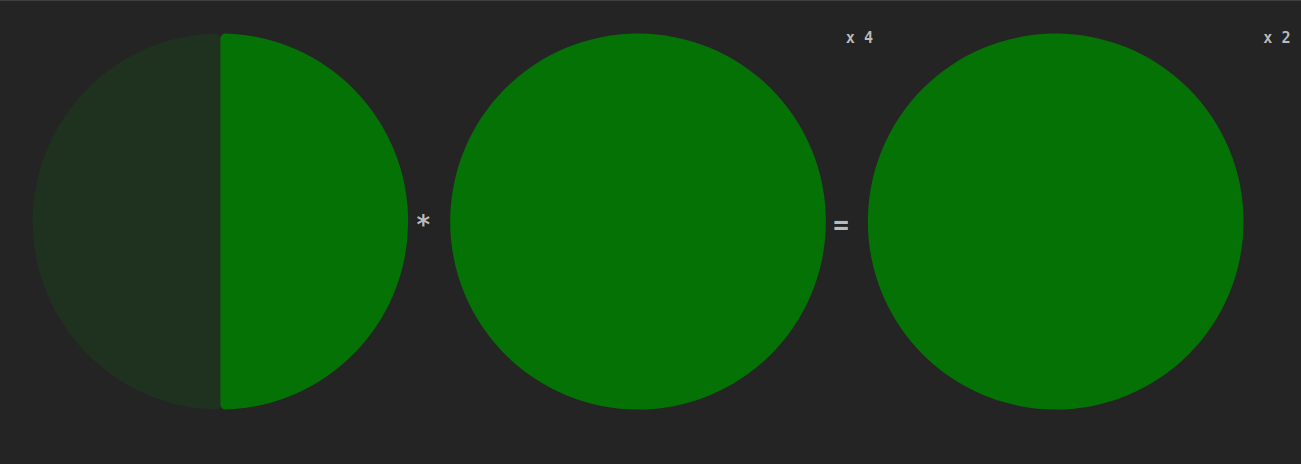
Multiplying a fraction is taking a fraction of a fraction. When we multiply a fraction, we cut the first fraction result by 'the second fraction denominator'. Then we take parts equal to the second fractions numerator.

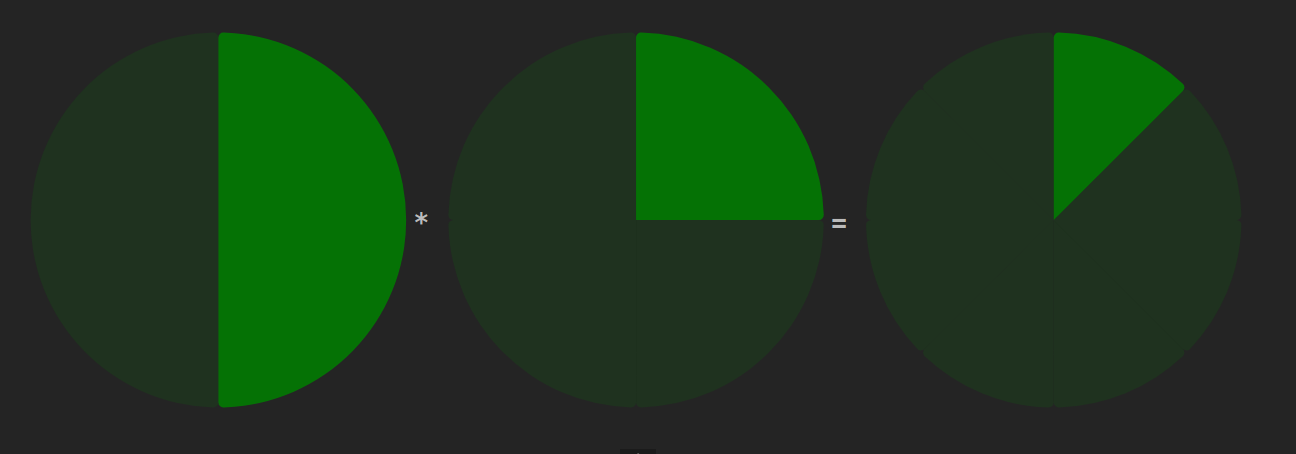
$$\frac{1}{2}*\frac{1}{4}=\frac{1}{8}$$ If we cut half of the whole into four parts : each part is now 1/8 of the whole.

Dividing

Why is the solution a bigger number than the fractions involved?

When we divide a fraction, we inverse the logic. We ask how much do we have in total if we have 'second fraction denominator' number of first fraction. Then we cut this amount into 'second fraction numerator' parts.

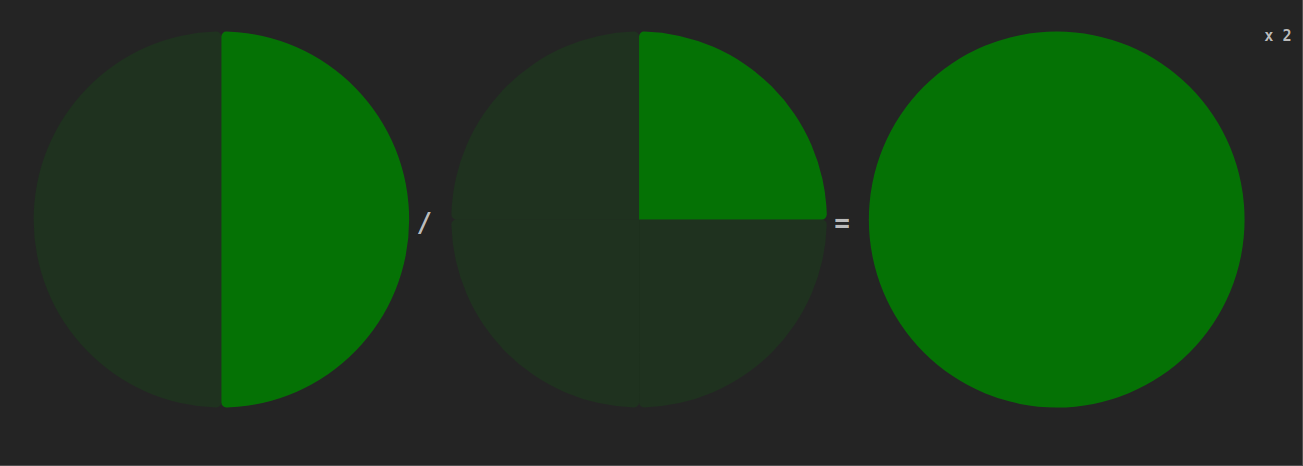
With this example: $$\frac{1}{2}/\frac{1}{4}=\frac{1}{2}*\frac{4}{1}=\frac{1*4}{2*1}=\frac{4}{2}=2$$

We took 4 times half of a pizza. It means we took 2 whole pizzas.